
Xiaojing (Ruby) Fu
Please visit my personal website here.
Current position: Miller Research Fellow at UC Berkeley (hosted by Department of Earth and Planetary Science and Prof. Michael Manga).
I obtained my PhD in Civil & Environmental Engineering from MIT in June 2017 with Prof. Ruben Juanes. Prior to my PhD, I received my MS in Computation for Design & Optimization from MIT in 2015 and BS in Applied Mathematics & Statistics from Clarkson University (Potsdam, NY) in 2011.
Publications
Pore-scale modeling of phase change in porous media;
L. Cueto-Felgueroso, X. Fu, R. Juanes; Physical Review Fluids, 3, 084302 (2018), doi:10.1103/PhysRevFluids.3.084302 [pdf].;Nonequilibrium thermodynamics of hydrate growth on gas–liquid interface;
X. Fu, L. Cueto-Felgueroso, R. Juanes; Phys. Rev. Lett.,120(14):144501 (2018).[pdf]
* Editor's suggestionViscous fingering with partially miscible fluids;
X. Fu, L. Cueto-Felgueroso, R. Juanes; Phys. Rev. Fluids, 2(10):104001 (2017).[pdf]
*Featured in APS 2018 calendar (month of February)Laboratory observations of the evolution and rise rate of bubbles with and without hydrate shells;
W. F. Waite, T. Weber, X. Fu, R. Juanes, C. Ruppel; Proceedings of the Ninth International Conference on Gas Hydrate (2017).Improved subsurface imaging of saline aquifers from transient pressure data during freshwater injection;
P. K. Kang, J. Lee, X. Fu, S. Lee, P. K. Kitanidis, and R. Juanes; Water Resour. Res., in press (2017). [pdf]Thermodynamic coarsening arrested by viscous fingering in partially miscible binary mixtures;
X. Fu, L. Cueto-Felgueroso, R. Juanes; Physical Review E, 94(3),033111(5) (2016). [pdf][video1][video2]Dissolution patterns from geochemical reactions during convection mixing in porous media;
X. Fu, L. Cueto-Felgueroso, D. Bolster, R. Juanes; Journal of Fluid Mechanics, 764:296-315 (2015). [pdf]Pattern formation and coarsening dynamics in three-dimensional convective mixing in porous media;
X. Fu, L. Cueto-Felgueroso, R. Juanes; Philosophical Transactions of the Royal Society A, 371:20120355 (2013). [pdf][videos]Understanding the impact of boundary and initial condition errors on the solution to a thermal diffusivity inverse Problem;
X. Fu, B. Leventhal, K. Fowler; SIAM Undergraduate Research Online, 4:S01123 (2011).[pdf]Uncertainty in modeled and observed climate change impacts on American Midwest hydrology;
J. M. Winter, P. J.-F. Yeh, X. Fu, E. A.B. Eltahir; Water Resources Research, 51(5):3635–3646 (2015). [link]Effects of model resolution on optimal design of subsurface flow and transport problems;
M.W.Farthing, K.R.Fowler, X. Fu, A.Davis, C.T.Miller; Advances in Water Resources, 38:27-37 (2012).[link]Experimental design of diffusion and desorption of contaminant in heterogeneous media;
G. Jiang, M. Crimi, K. Fowler, X. Fu; Water Science & Technology, 64(4):988-998 (2011). [link]Parameter Identification and Sensitivity Analysis to a Thermal Diffusivity Inverse Problem;
B. Leventhal, X. Fu, K. Fowler, O. Eslinger; Involve, 8(3) (2015).[link]
Nonequilibrium thermodynamics of hydrate growth on a gas-liquid interface
Methane hydrate is an ice-like solid that forms out of an aqueous solution of water and dissolved methane under moderate pressure and low temperature conditions. A large body of work over the past few decades has established a fundamental understanding of methane hydrate thermodynamics. In this work, we develop a continuum-scale phase-field model to study gas–liquid–hydrate systems far from thermodynamic equilibrium. We design a Gibbs free energy functional for methane–water mixtures that recovers the isobaric temperature–composition phase diagram under thermodynamic equilibrium conditions. The proposed free energy is incorporated into a phase-field model to study the dynamics of hydrate formation on a gas–liquid interface. We elucidate the role of initial aqueous concentration in determining the direction of hydrate growth at the interface, in agreement with experimental observations. Our model also reveals two stages of hydrate growth at an interface—controlled by a crossover in how methane is supplied from the gas and liquid phases—which could explain the persistence of gas conduits in hydrate-bearing sediments and other nonequilibrium phenomena commonly observed in natural methane hydrate systems.

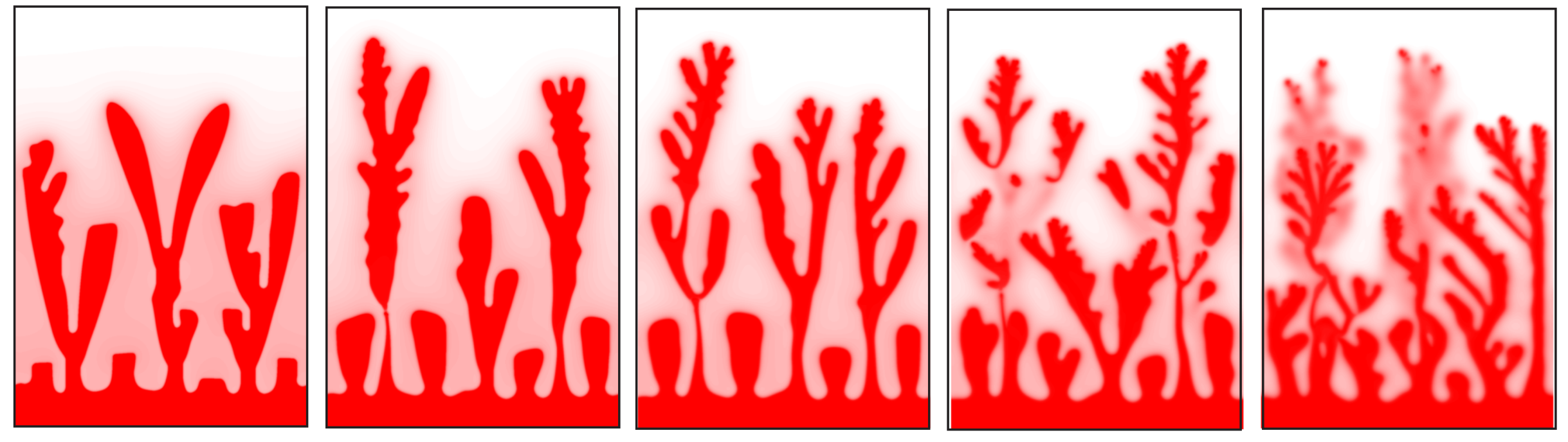
Viscous fingering with partially miscible fluids
Viscous fingering–the fluid-mechanical instability that takes place when a low- viscosity fluid displaces a high-viscosity fluid–has traditionally been studied under either fully miscible or fully immiscible fluid systems. Here we study the impact of partial miscibility (a common occurrence in practice) on the fingering dynamics. Through a careful design of the thermodynamic free energy of a binary mixture, we develop a phase-field model of fluid-fluid displacements in a Hele-Shaw cell for the general case in which the two fluids have limited (but nonzero) solubility into one another. We show, by means of high-resolution numerical simulations, that partial miscibility exerts a powerful control on the degree of fingering: fluid dissolution hinders fingering while fluid exsolution enhances fingering. We also show that, as a result of the interplay between compositional exchange and the hydrodynamic pattern-forming process, stronger fingering promotes that the system approach thermodynamic equilibrium faster.
Thermodynamic coarsening arrested by viscous fingering in partially miscible binary mixtures
In this work, we study the evolution of binary mixtures far from equilibrium, and show that the interplay between phase separation and hydrodynamic instabilities can arrest the Ostwald ripening process characteristic of non-flowing mixtures. We describe a model binary system in a Hele-Shaw cell using a phase-field approach with explicit dependence of both phase fraction and mass concentration. When the viscosity contrast between phases is large (as is the case for gas and liquid phases), an imposed background flow leads to viscous fingering, phase branching and pinch-off. This heterogeneous and dynamic flow limits phase growth and arrests thermodynamic coarsening. As a result, the system reaches a regime of statistical steady state in which the binary mixture is permanently driven away from equilibrium.
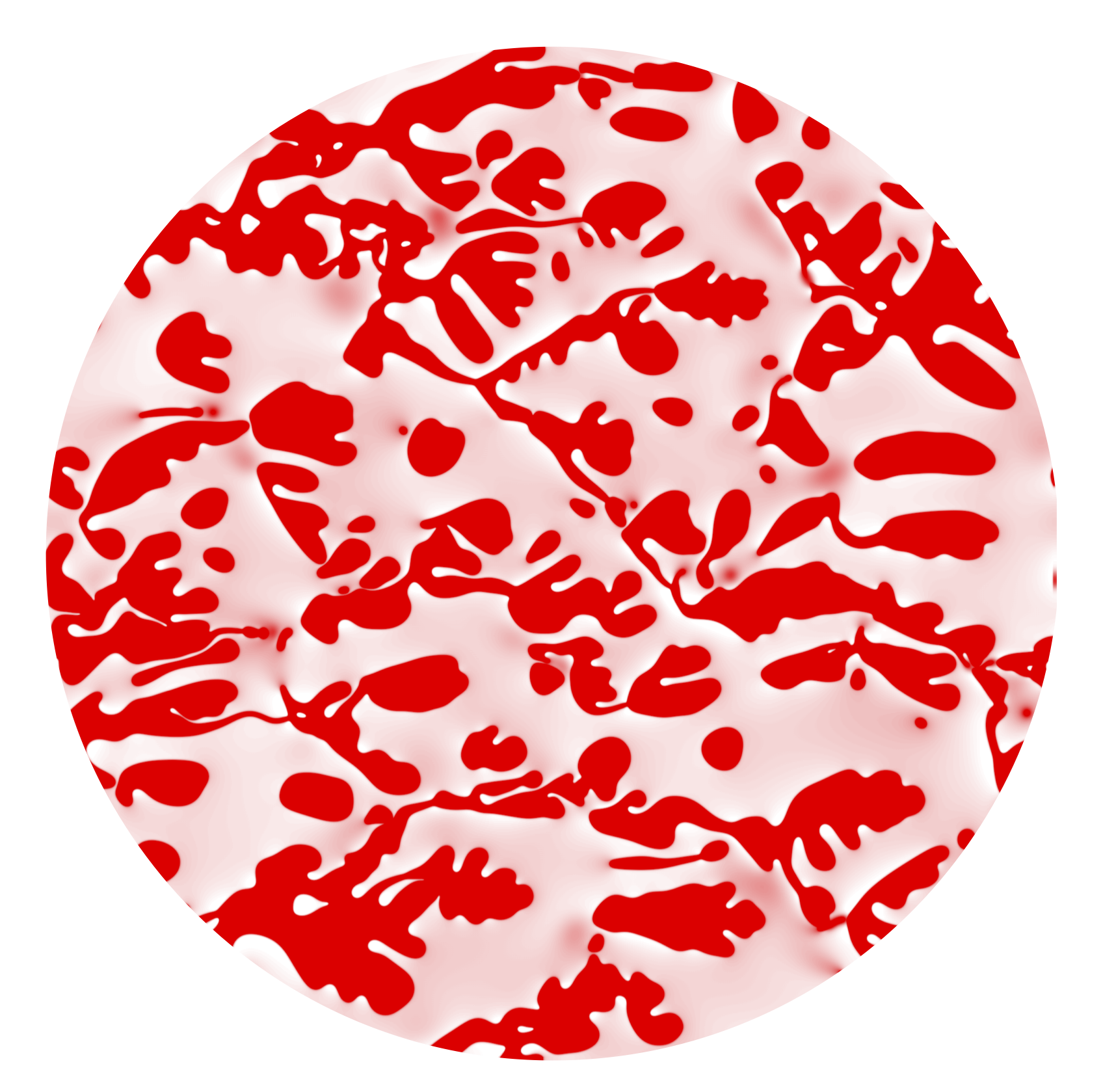
Video 1 (Ostwald ripening in two-component two-phase mixture) : This is a numerical simulation describing the process of nucleation and coarsening of gas bubbles (red) out of a liquid (pink) that is initially supersaturated.
Video 2 (Arrest of Ostwald ripening under viscous fingering): This is a numerical simulation showing the dynamic interactions amongst gas bubbles (red) dispersed in a more viscous liquid (pink) when the mixture is driven under a cyclic background flow. The gas bubbles are first nucleated without background flow until t=40. Once flow is introduced at t=40, the gas-liquid displacement is destabilized by a viscosity contrast of 148.
Rock dissolution patterns and geochemical shutdown of CO2–brine–carbonate reactions during convective mixing in porous media
Motivated by the process of CO2 convective mixing in porous media, here we study the formation of rock-dissolution patterns that arise from geochemical reactions during Rayleigh--Bénard--Darcy convection. Under the assumption of instantaneous chemical equilibrium, we adopt a formulation of the local reaction rate as a function of scalar dissipation rate---a measure that depends solely on flow and transport---and chemical speciation, which is a measure that depends only on the equilibrium thermodynamics of the chemical system. We use high-resolution simulations to examine the interplay between the density-driven hydrodynamic instability and the rock dissolution reactions, and analyze the impact of geochemical reactions on the macroscopic mass exchange rate. We find that dissolution of carbonate rock initiates in regions of locally high mixing, but that the geochemical reaction shuts down significantly earlier than shutdown of convective mixing. This early shutdown feature reflects the important role that chemical speciation plays in this hydrodynamics--reaction coupled process. Finally, we extend our analysis to three dimensions and explore the morphology of dissolution patterns in 3D.
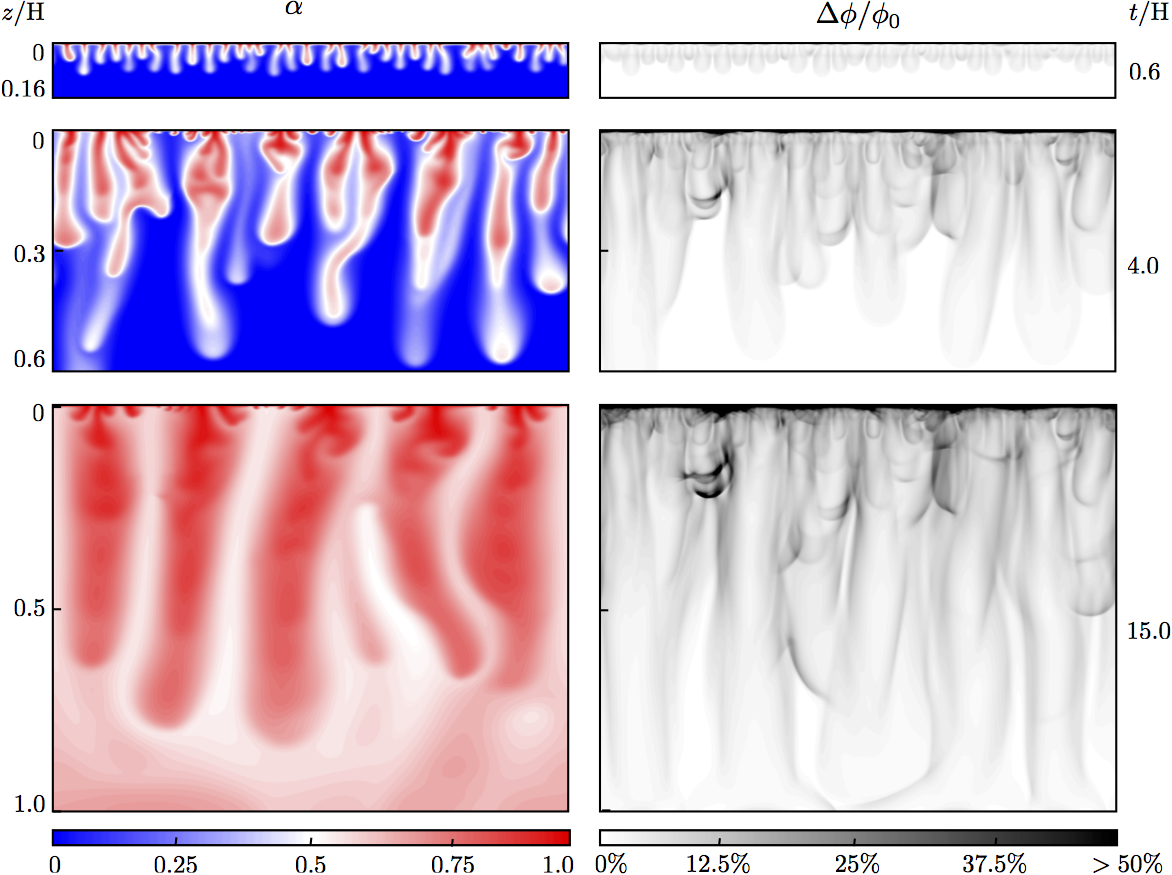
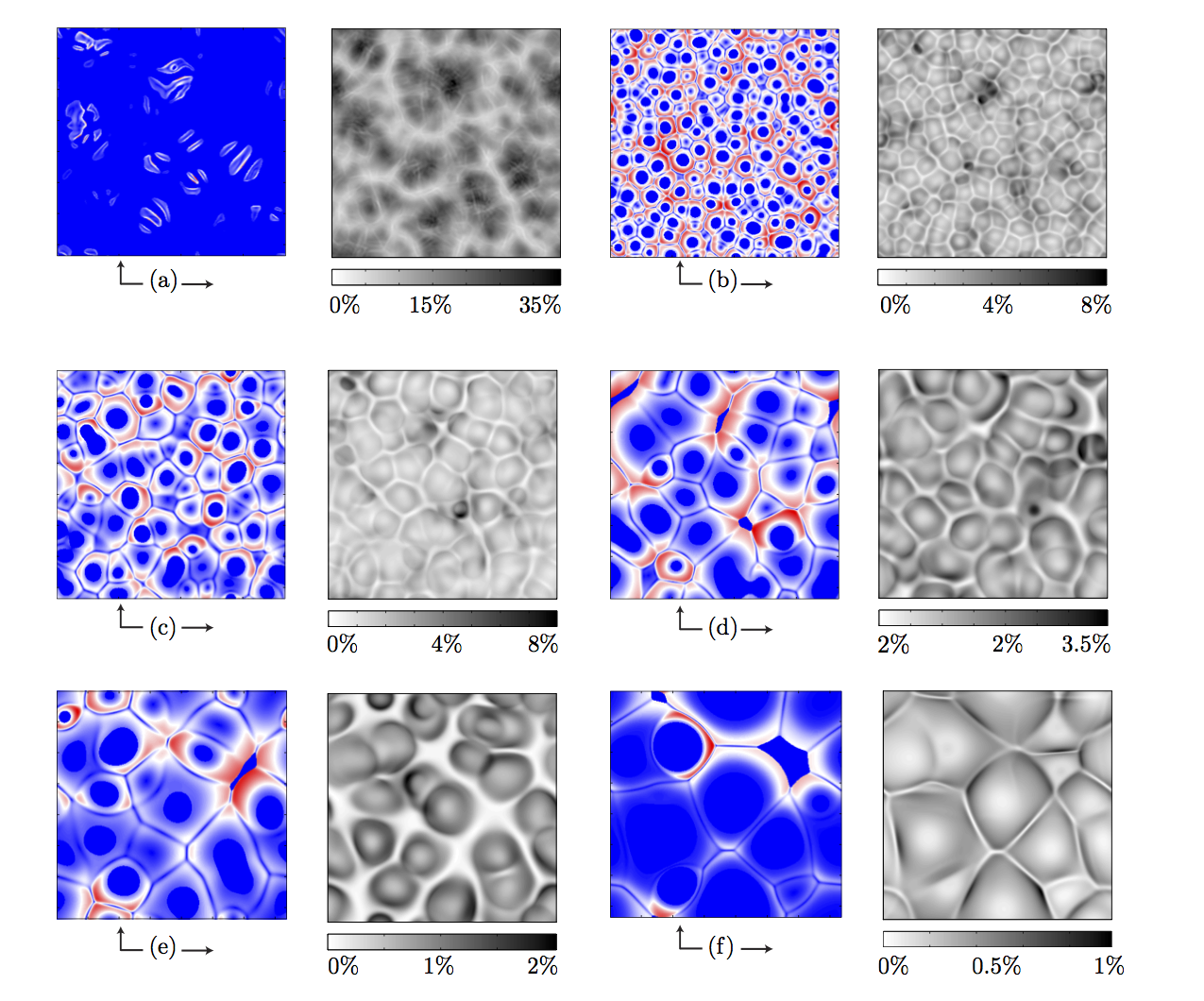
Pattern formation and coarsening dynamics in three-dimensional convective mixing in porous media
Geologic carbon dioxide sequestration entails capturing and injecting CO2 into deep saline aquifers for long-term storage. The injected CO2 mixes with groundwater, forming a mixture that is denser than the initial groundwater. The local increase in density triggers a gravitational instability at the boundary layer that further develops into columnar plumes of CO2-rich brine, a process that greatly accelerates solubility trapping of the CO2. Here, we investigate the pattern-formation aspects of convective mixing during geological CO$_2$ sequestration by means of high-resolution three-dimensional simulation. We find that the CO2 concentration field self-organizes as a cellular network structure in the diffusive boundary layer at the top boundary. By studying the statistics of the cellular network, we identify various regimes of finger coarsening over time, the existence of a nonequilibrium stationary state, and a universal scaling of 3D convective mixing.
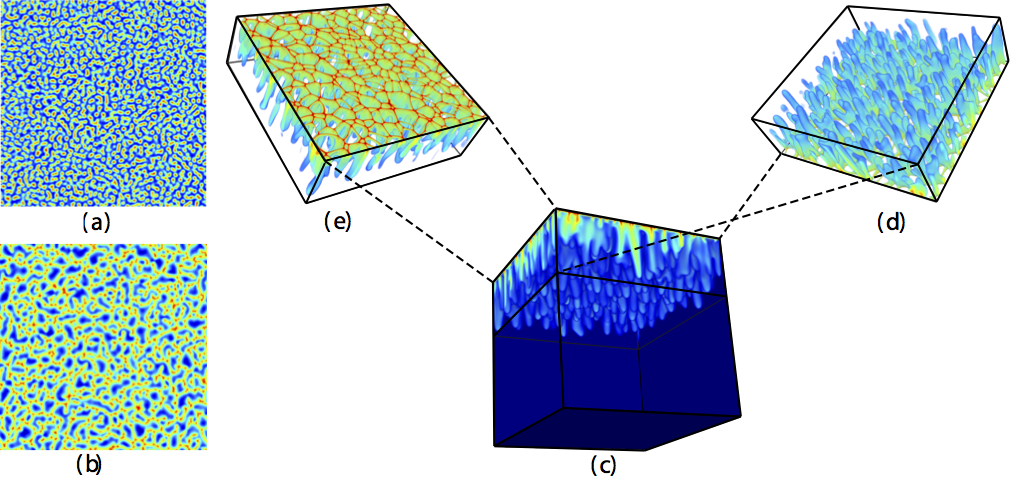
Supplementary Video 1: Video showing the evolution of the 3D convective mixing pattern from two different angles, corresponding to a Rayleigh number Ra=6400, computed on a grid of 512-cube.
Supplementary Video 2: Video showing the evolution of the emerging patterns in the diffusive boundary layer at the top boundary, for a Rayleigh number Ra=6400, computed on a grid of 512-cube.
=== Fluid instability and foams in an Espresso ===

77 Mass. Ave., Room 1-350
Cambridge, MA 02141, USA
email: rubyfu@mit.edu