
Bauyrzhan Primkulov
Please visit my personal website here.
I am a Ph.D. candidate in the Department of Civil and Environmental Engineering. Interfacial tension dominated flows and applied mathematics are two fields that keep me a happy and hungry student.
Publications
Avalanches in Strong Imbibition;
B.K. Primkulov, B. Zhao, C.W. MacMinn, and R. Juanes; submitted.Wettability and Lenormand's diagram;
B.K. Primkulov, A.A. Pahlavan, X. Fu, B. Zhao, C.W. MacMinn, and R. Juanes; Journal of Fluid Mechanics 923, A34 (2021) [pdf]Photoporomechanics: An Experimental Method to Visualize the Effective Stress Field in Fluid-Filled Granular Media;
W. Li, Y. Meng, B. K. Primkulov, and R. Juanes; Physical Review Applied 16 (2), 024043 (2021) [pdf]Multiphase flow and granular mechanics;
R. Juanes, Y. Meng, and B. K. Primkulov; Physical Review Fluids, 5 (11), 110516 (2020) [pdf]Characterizing dissipation in fluid–fluid displacement using constant-rate spontaneous imbibition;
B.K. Primkulov, J. Y. Y. Chui, A. A. Pahlavan, C. W. MacMinn, and R. Juanes; Physical Review Letters, 125 (17), 174503 (2020) [pdf]Jamming transition and emergence of fracturing in wet granular media;
Y. Meng, B. K. Primkulov, Z. Yang, C. Y. Kwok, and R. Juanes; Physical Review Research, 2, 022012 (2020) [pdf]Spin coating of capillary tubes;
B.K. Primkulov, A.A. Pahlavan, L. Bourouiba, J.W.M. Bush and R. Juanes; Journal of Fluid Mechanics, 886, A30 (2020) [pdf]Signatures of fluid-fluid displacement in porous media: wettability, patterns, and pressures;
B.K. Primkulov, A.A. Pahlavan, X. Fu, B. Zhao, C.W. MacMinn and R. Juanes; Journal of Fluid Mechanics, 875, R4 (2019) [pdf]Comprehensive comparison of pore-scale models for multiphase flow in porous media;
B. Zhao, C.W. MacMinn, B.K. Primkulov, Y. Chen, A.J. Valocchi, J. Zhao, Q. Kang, K. Bruning, J.E. McClure, C.T. Miller, A. Fakhari, D. Bolster, T. Hiller, M. Brinkmann, L. Cueto-Felgueroso, D.A. Cogswell, R. Verma, M. Prodanovic, J. Maes, S. Geiger, M. Vassvik, A. Hansen, E. Serge, R. Holtzman, Z. Yang, C. Yuan, B. Chareyre, R. Juanes; Proceedings of the National Academy of Sciences, p.201901619 (2019) [pdf]Quasistatic fluid-fluid displacement in porous media: Invasion-percolation through a wetting transition;
B.K. Primkulov, S. Talman, K. Khaleghi, A.R. Shokri, R. Chalaturnyk, B. Zhao, C.W. MacMinn, and R. Juanes; Physical Review Fluids, 3(10), p.104001 (2018) [pdf]3D printed sandstone strength: curing of furfuryl alcohol resin-based sandstones;
B.K. Primkulov, J. Chalaturnyk, R. Chalaturnyk, G. Zambrano; 3D Printing and Additive Manufacturing, 4(3), pp.149-156 (2017) [pdf]Impact of salinity on warm water‐based mineable oil sands processing;
T. Chen, F. Lin, B.K. Primkulov, L. He and Z. Xu; The Canadian Journal of Chemical Engineering, 95(2), pp.281-289 (2017) [pdf]Microscale liquid-liquid displacement dynamics: Molecular kinetic or hydrodynamic control;
B.K. Primkulov, F. Lin and Z. Xu; Colloids and Surfaces A: Physicochemical and Engineering Aspects, 497, pp.336-343 (2016) [pdf]Dewetting dynamics of a solid microsphere by emulsion drops;
F. Lin, L. He, B.K. Primkulov and Z. Xu; The Journal of Physical Chemistry C, 118(25), pp.13552-13562 (2014) [pdf]
Multiphase Flow in Porous Media
Many natural and engineered processes involve one fluid displacing another in porous media. However, a full understanding of how porous material properties, such as wettability, influence the formation of patterns (and thus the efficiency of such displacement) has not yet been established. We aim to fill this gap through theoretical modeling and experiments.
Quasistatic fluid-fluid displacement in porous media: Invasion-percolation through a wetting transition
We first developed a model that, through pore-scale displacement mechanisms, captures the fluid-fluid front patterns in a quasi-steady regime. As the porous media wettability changes from strong drainage to strong imbibition, the model naturally crosses over from invasion-percolation to cooperative filling and corner flow (a mechanism that has eluded many of the models until now).
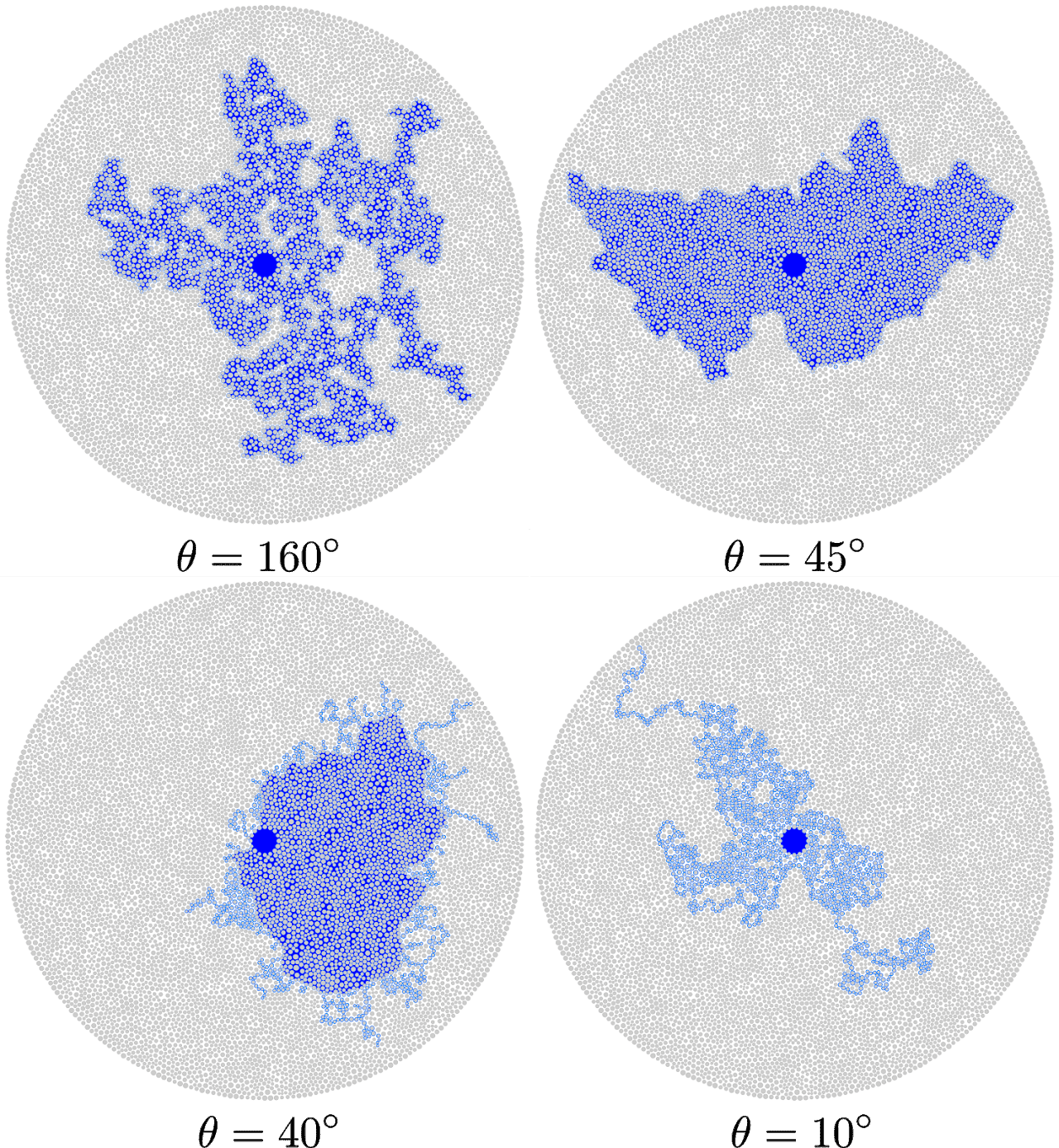
Signatures of fluid–fluid displacement in porous media: wettability, patterns and pressures
We then extended our fluid-fluid displacement model to arbitrary capillary numbers through analogy with an electric circuit, where resistors captured viscous effects while a combination of a capacitor and a battery reproduced the behavior of a meniscus in a pore throat. This allowed examining the signatures of the fluid-fluid displacement regimes (viscosity or capillary dominated) through both macroscopic displacement patterns and injection pressure signal alone. The latter is of particular importance since the pressure signal is often the only source of information in natural porous materials.
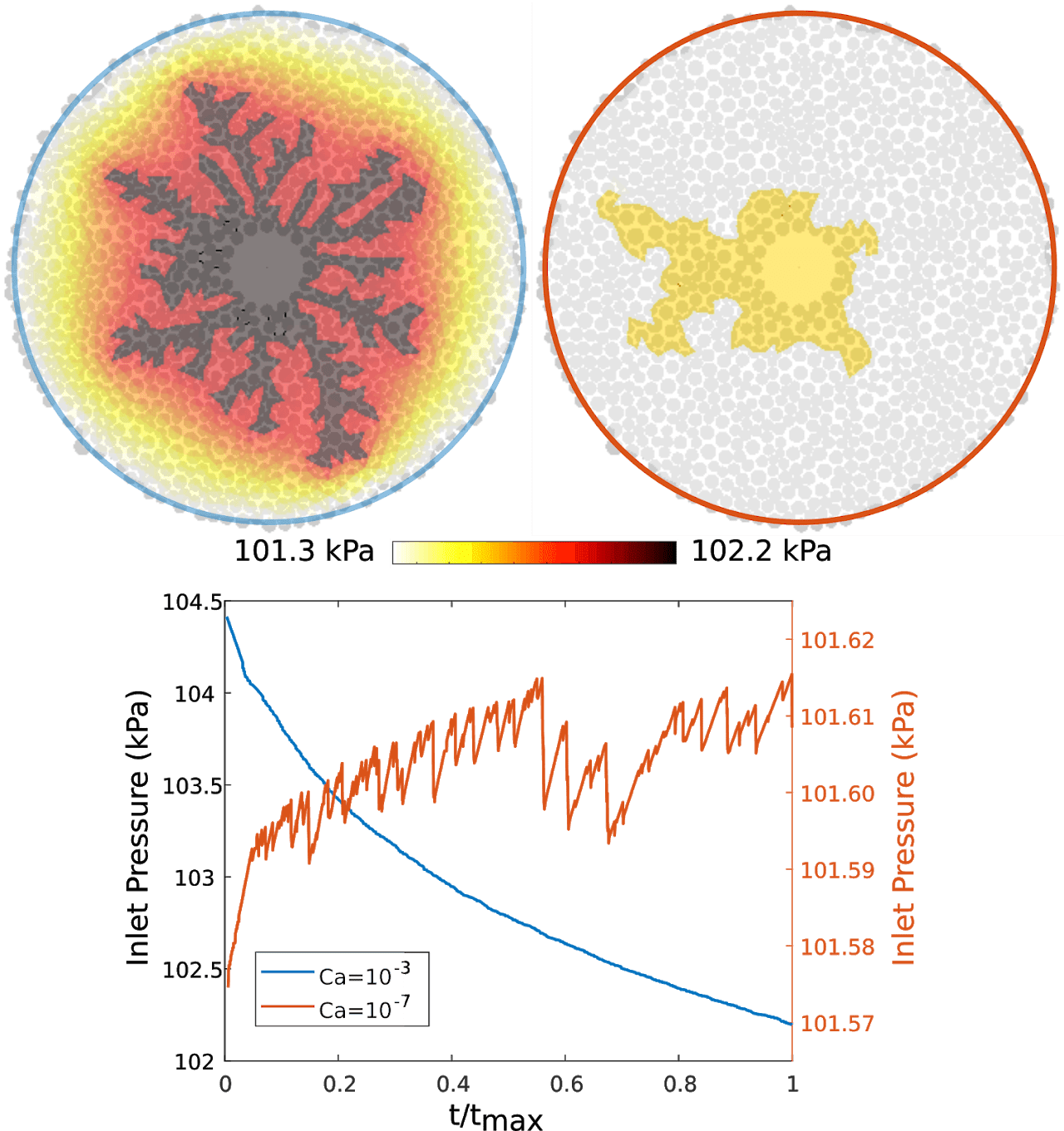
Comprehensive comparison of pore-scale models for multiphase flow in porous media
We then perform an objective comparison of a variety of state-of-the-art pore-scale models, including lattice Boltzmann, stochastic rotation dynamics, volume-of-fluid, level-set, phase-field, and pore-network models. We compare the results of the 14 participating teams both qualitatively and quantitatively using several standard metrics, such as fractal dimension, finger width, and displacement efficiency. We find that no single method excels across all conditions and that thin films and corner flow present substantial modeling and computational challenges.

Wettability and Lenormand's diagram
Fluid–fluid displacement in porous media has been viewed through the lens of Lenormand's phase diagram since the late 1980s. This diagram suggests that the character of the flow is controlled by two dimensionless parameters: the capillary number and the viscosity ratio. It is by now well known, however, that the wettability of the system plays a key role in determining the pore-scale displacement mechanisms and macroscopic invasion patterns. Here, we endow Lenormand's diagram with the impact of wettability using dynamic and quasi-static pore-network models. By using the fractal dimension and the ratio of characteristic viscous and capillary pressures we delineate the five principal displacement regimes within the extended phase diagram: stable displacement, viscous fingering, invasion percolation, cooperative pore filling and corner flow. We discuss the results in the context of pattern formation, displacement-front dynamics, pore-scale disorder and displacement efficiency.
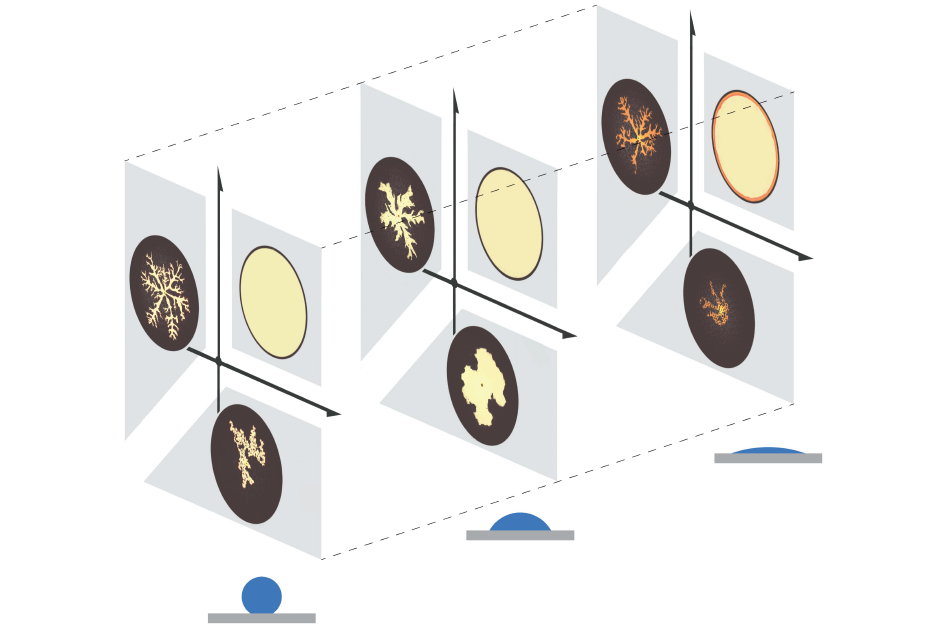
Interfacial Fluid Dynamics
The dynamics of fluid-fluid interfaces has direct relevance to many industrial and manufacturing applications, such as printing, coating, detergency, oil recovery, and mineral processing. Yet, this field is abundant in unsolved fundamental problems. We aim to uncover the physical mechanisms at play in wetting dynamics and free-boundary problems with theory and experiments.
Characterizing dissipation in fluid–fluid displacement using constant-rate spontaneous imbibition
When one fluid displaces another in a confined environment, some energy is dissipated in the fluid bulk and the rest is dissipated near the contact line. Here we study the relative strengths of these two sources of dissipation with a novel experimental setup: constant-rate spontaneous imbibition experiments, achieved by introducing a viscous oil slug in front of the invading fluid inside a capillary tube. We show that a large fraction of dissipation takes place near the contact line, and rationalize the observations by means of a theoretical analysis of the dynamic contact angles of the front and back menisci of the oil slug—a result that bears important implications for macroscopic descriptions of multiphase flows in microfluidic systems and porous media.
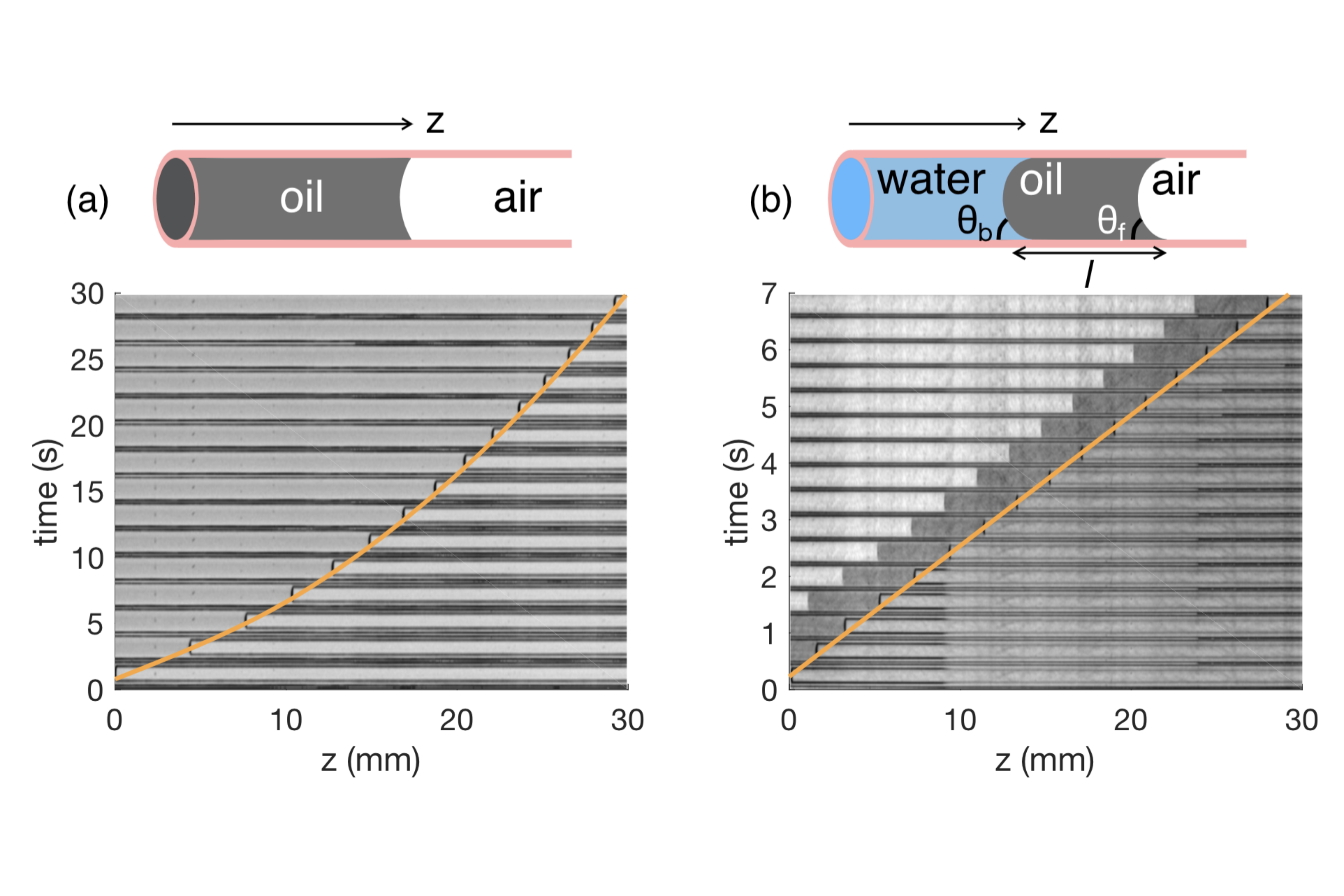
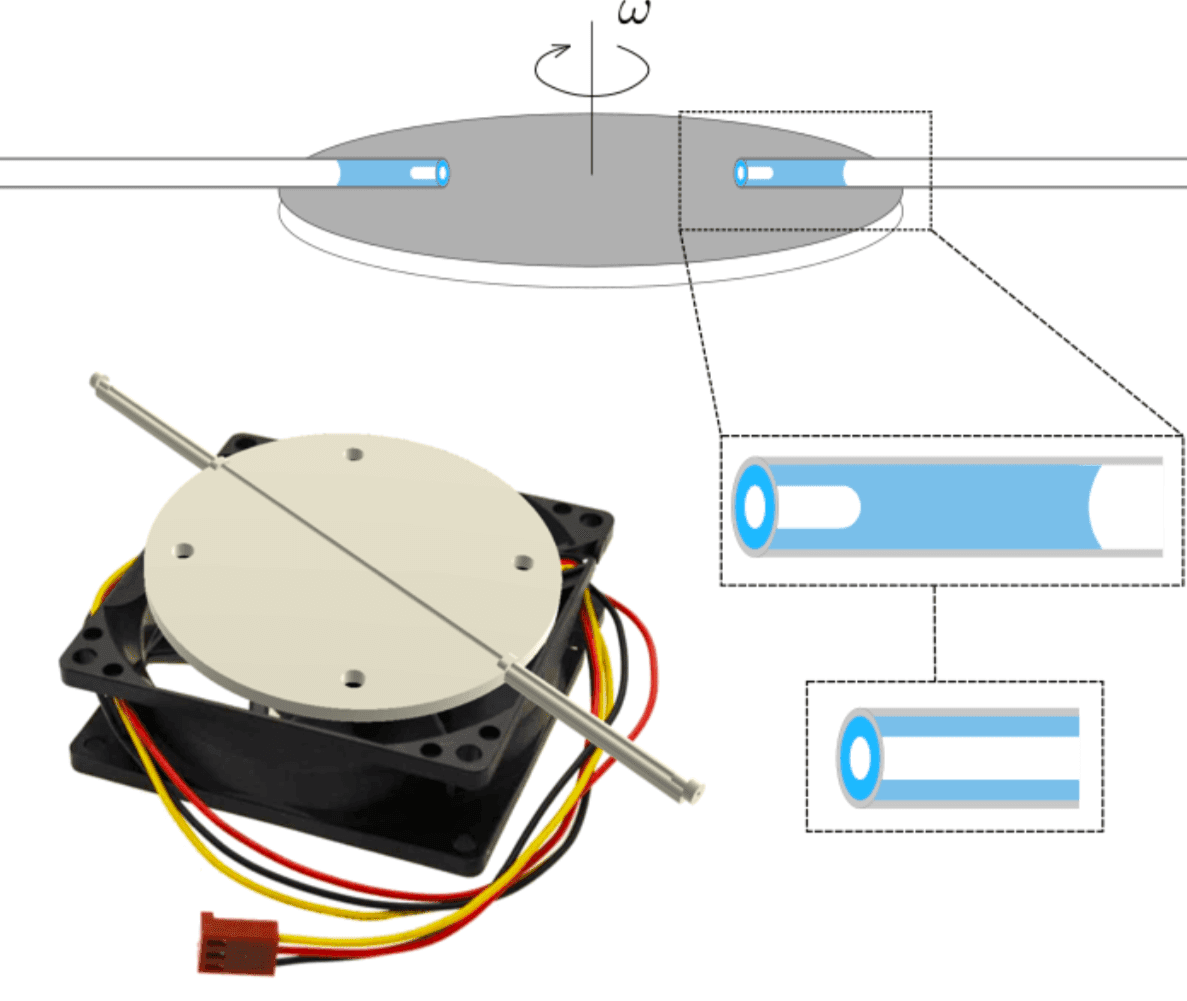
Spin coating of capillary tubes
We present the results of a combined experimental and theoretical study of the spin-coating of the inner surface of capillary tubes with viscous liquids, and the modified Rayleigh-Plateau instability that arises when the spinning stops. We show that during the spin-coating, the thinning of the film is governed by the balance of viscous and centrifugal forces, resulting in the film thickness scaling as h ~ t^{-1/2}. We demonstrate that the method enables one to reach uniform micrometer-scale films on the tube walls. Finally, we discuss potential applications with curable polymers that enable precise control of film geometry and wettability.
Dept. of Civil and Environmental Engineering
Massachusetts Institute of Technology
77 Massachusetts Ave, Room 1-350
Cambridge, MA 02139, USA
Email: bprimkul@mit.edu